Introduction
The Steemit platform offers us the unique opportunity to craft educational material that is: 1. easily accessible to anyone who wishes to view it. 2. Free and open for all to read and learn from. There is no subscription fee, and no expensive text book purchase necessary. So long as someone has the initiative, creativity, and knowledge to put together an educational series, it is possible to increase the general knowledge of a large number of people. As I feel that the future of this platform is extremely bright and the number of those who will come in contact with the material is large, I have been writing blog pieces with the sole purpose of educating the reader.
I will continue to do that here with this series, where I will attempt to slowly compile together the material taught in an undergraduate level general chemistry course to provide free and easy access to better understanding of chemistry. I taught undergraduate chemistry (as a graduate assistant) for a number of years and I hope to instill in each of you who read this and the following posts, an increased understanding of chemistry and a greater appreciation for the complexities of the world in which we live.
Chemistry and the Scientific Method
The foundation upon which all scientific disciplines are developed is the core approach to research called the scientific method. The initial step of the scientific method is to identify a Problem. With a problem in mind a researcher then proposes a Hypothesis (a potential explanation for your initial observation aka your initial explanation of the problem) and carefully Designs Experiments in order to test the validity of that hypothesis. The experiments proposed can be either qualitative (designed to describe something generally) or quantitative (a numerical description of a result obtained by making measurements) both of these experimental methods are equally good at generating information and can aide in confirming or denying your hypothesis. Having obtained experimental data, the researcher then Interprets that data and determines whether their hypothesis is confirmed or not. At no point in the scientific method should a researcher design experiments to prove their hypothesis correct, science is not about being right from the outset, it is about determining what is correct through well designed experiments!
Once researchers have collected a significant amount of data and confirmed or denied many hypotheses the information learned is summarized into two possible forms. The first is a Theory, which is an idea which unites together a bunch of facts or observations. A theory is only as good as the level of scrutiny it can withstand, so every theory should constantly be tested by further research and new hypotheses. Scientific theories are not definitive facts, however they are the best determined explanation for a given set of observations. The second possible form for a summary of learned information is a Law. A scientific law is a verbal or mathematical representation of a relationship between two or more things that is always the same under a given set of conditions. What this means is, if people make measurements under the same conditions to measure a specific phenomenon and everyone always comes up with the same exact result, we can make a law describing that observation as it is always true. An example of a scientific law is the first law of thermodynamics. It states this: ΔU = Q + W, where ΔU is the change in internal energy of something, Q is the heat supplied to the system and W is the work performed by the system. Most hypotheses do not lead to the generation of theories or laws and theories do not necessarily lead to identifying laws. In fact theories don’t always come before laws, nor do laws always come before theories; unfortunately science just doesn’t happen in an easy to list stepwise fashion.
What is Chemistry?
Chemistry is the study of matter and the changes that happen to matter. Matter is anything that takes up space and possesses Mass. Mass is specifically the weight attributed to a specific amount of something/anything. Matter has 3 forms Solid, Liquid and Gas. Solids have defined shapes, liquids do not have defined shapes and thus are able to flow but cannot indefinitely expand, and gasses have no specific volume or shape and can expand indefinitely.
Figure 1: The Three States of Matter

Source
We can break matter down into two big categories, Substances and Mixtures. A substance is something with a constant composition. A mixture is any combination of two or more structures that can be separated back out into its constituent parts. Substances can be further subdivided into two more types, Elements and Compounds. Elements are substances which can’t be separated into anything simpler by chemical means (they are the substances of the periodic table aka Oxygen, Nitrogen, Sodium, Platinum etc. see Reference Figure: Periodic Table), while compounds are substances composed of two or more elements, in specific and unchanging proportions (for example the alcohol we drink… ethanol, C2H6O all ethanol is composed of 2 Carbons, 6 Hydrogens and 1 Oxygen no matter what its source is).
Measurements
Most if not all chemistry experiments involve some sort of measurement. Often times many properties of matter are measured (temperature, mass, volume, color etc.) as a chemical reaction proceeds or at the end of a reaction. In order to standardize the way in which measurements are taken a standard set of units were devised called SI (which stands for System International d’Unites, because it was devised by French scientists).
Table 1: Examples of Common SI Units
| Measured Quantity | Unit Name | Symbol Used |
|---|---|---|
| Mass | Kilogram | kg |
| Temperature | Kelvin | K |
| Time | Second | s |
| Length | Meter | m |
| Amount of a Substance | mole | mol |
There are also a variety of prefixes used to aide quickly quantifying things in various factors of 10
| Prefix | Symbol | Amount |
|---|---|---|
| Giga | G | 1000000000 |
| Mega | M | 1000000 |
| Kilo | k | 1000 |
| Deci | d | 10 |
| Centi | c | 0.1 |
| Mili | m | 0.001 |
| Micro | µ | 0.000001 |
Scientific Notation
Having established some of the general units and prefixes used in chemistry, now let us discuss how scientists deal with the sometimes very large and very small numbers. Say we were dealing with the mass of the earth for a calculation (because we are ballers and we do reactions on an Earth scale) Rather than writing a very large number 5972000000000000000000000 kg (which is the actual mass of the earth) we can move the decimal place from the right to the left and multiply by a factor of 10. We end up with notation looking like this 5.92x1024 kg, or 5.92 times (10x10…x10) for a total of 24 multiplications of 10 (I know you understand this, it is just for completions sake). It is much easier to deal with large or small numbers in the format of scientific notation then it is writing out all of those zeros.
Doing Math with Scientific Notation
Addition
To add numbers together in scientific notation simply add the non 10 number together:
5.0x104 + 5.0x104 = 10.0x104 and adjust the factor of 10 accordingly = 1.0x105.
Subtraction
To subtract numbers in scientific notation simply subtract the non 10 number
5.0x104- 2.0x104 = 3.0x104
Multiplication
To multiply numbers in scientific notation, multiply the number then add the superscript of the factor of 10 together
5.0x104 x 5.0x104= 25.0x10(4+4) = 25.0x108 and adjust the factor of 10 accordingly = 2.5x109
Division
To divide numbers in scientific notation, divide the number then subtract the superscript of the factor of 10 from one another.
(5.0x104 )/(2.5x102) = 2.0x10(4-2) = 2.0x102 and adjust the factor of 10 accordingly (here it is correct).
The Bane of Every Scientists Existence: Significant Figures
Finally we discuss significant figures which are the number of digits in a given number that actually mean something (I will explain). In order to talk about this we must first define Accuracy and Precision. Accuracy refers to your ability to measure what you expect, while precision is being able to reproduce your result. For an example of these two terms see Figure 2 (shooting the bullseye), here we can see an example of precision (three shots clustered together), but no accuracy (not close to the bullseye). We see accurate (all close to the bullseye) but no precision (they are scattered far apart from one another. Then finally we have the holy grail of science both accuracy and precision (or in this case, a good shooter who can reproducibly hit that bullseye).
Figure 2: Accuracy Vs. Precision
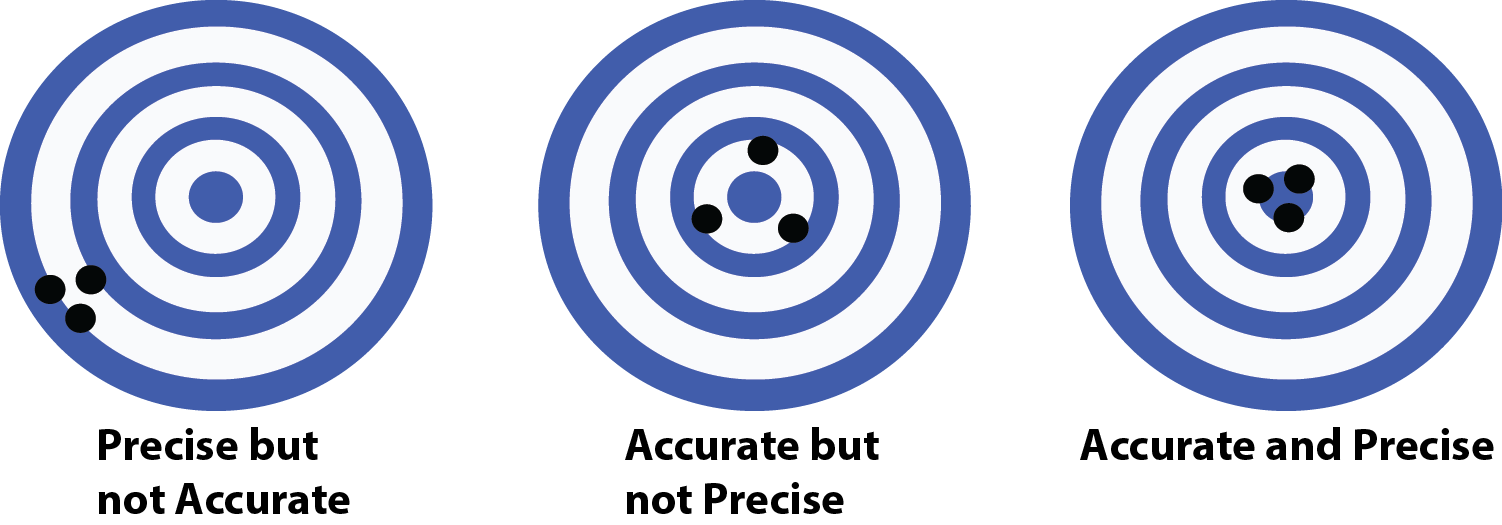
Source: Self
Significant figures are largely a measure of the degree to which a given measurement can be accurate. If I told you I went to a scale and massed out 25.6578400298367283058737820293775500239488239947756 g of Sodium Chloride (NaCl, table salt) you would tell me I was a dumbass because there is no way that scale has the accuracy to determine that many decimal places. For this we would say a scale is accurate to four significant figures (or two decimal places), thus I could only reasonably say I massed out 25.66 g of the NaCl. As for most things in science, people got together and figured out a bunch of rules for significant figures…
Rules for determining significant figures:
1.) Any non-zero non decimal number is significant. Eg: 1234 has four significant figures, while 1230 has only three. (Technically it may still have four as zeros to the right may or may not be significant… it is possible to mass out exactly 20 g of something for instance, there the zero is significant).
2.) Zeros inside of two or more non zero numbers ARE always significant: 1204 has four significant figures, while 1200 has two (or four for the same reason discussed above, it is difficult to know from the context of this example).
3.) For a decimal number zeros to the left of non-zero numbers are NOT significant: 0.000123 has three significant figures, while 0.0000000000000000000123 still has three significant figures.
4.) All zeros to the right of a number with a decimal are significant: 1.0 has two significant figures, a separate example 0.0000123 has three significant figures but 0.00001230 has four. One final example 1.002 has four significant figures.
5.) Scientific notation tells us exactly how many significant figures we have all digits in the main number are significant: 5.0x105 has two significant figures while 5.00x105 has three.
These rules allow us to know how many digits to record for a given measurement, given the accuracy of the device we are using.
Future Posts
This post summed up most of the material which would be covered in the first few introductory classes of a general chemistry course. Subsequent posts will cover: additional chemical properties (density, temperature and mass calculations), What are Atoms and Molecules?, Chemical Reactions, The Periodic Table, Electronic Configuration of Atoms, Chemical Bonding, and Molecular Geometry.
Reference Figure: Periodic Table
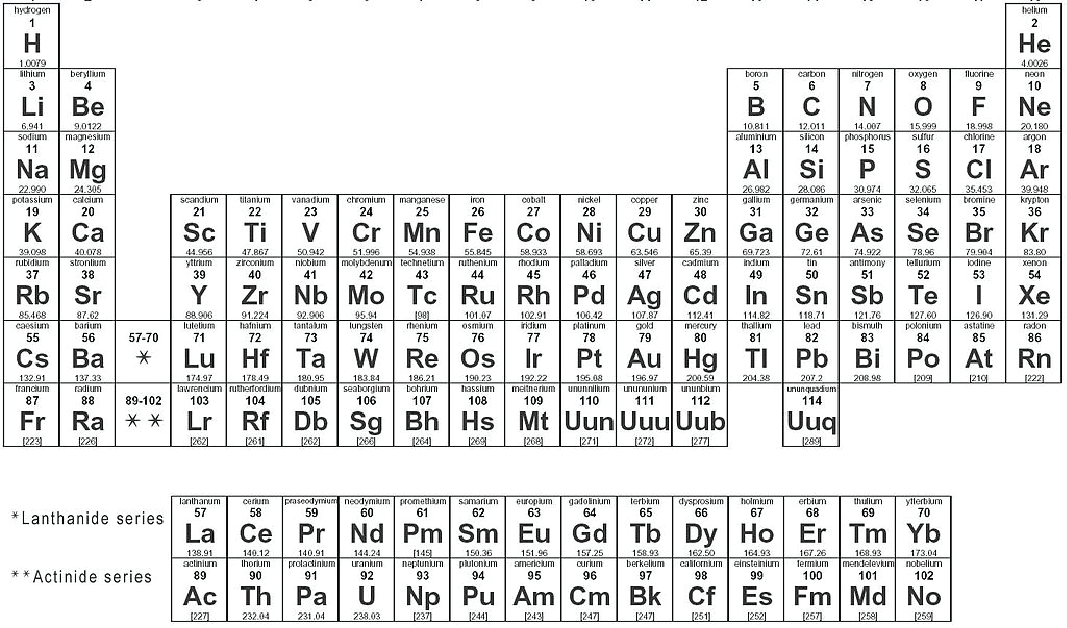
Source
If you like my work, please consider giving me a follow: steemit.com/@justtryme90. I am a PhD holding biochemist with a love for science. My future science blog posts will cover a range of topics in the biology/chemistry fields.
Thank you for your support of my work!
Any updates to this document have been to improve grammatical errors or sentence flow for ease of understanding.

