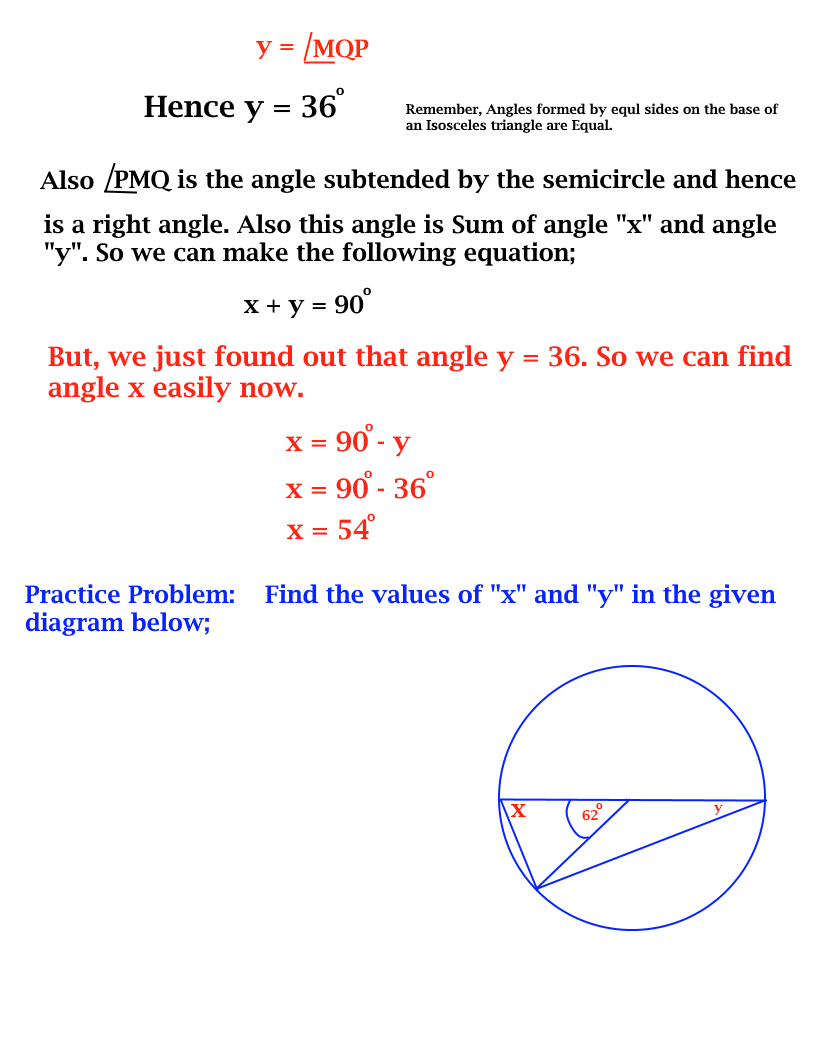
Finding the missing angles in a circle using different properties of circles
We will use all the basic circle terminology and the circle properties we discussed in the previous posts to solve the problems.
Keep in mind the the following terms about circles:
- Tangent is a line segment which intersect a circle only at one point.
- The radius and the tangent make a right angle at the point of a tangency.
- Any line segment intersecting a circle at two points is known as the chord of the circle.
- The diameter of the circle is its longest chord.
- If the radius of the circle intersect a chord at right angle then the radius bisects the chord.
- If the radius bisects the chord then it is perpendicular to the chord (reverse of the above property)
- Now the perpendicular bisector of the chord of the circle passes though its radius (both the above properties)
- Central angle of a circle is twice the inscribed angle subtended by the same arc which is making the central angle.
- Angle subtended by a semicircle is a right angle.
Trust me I have forgotten all the above before starting to write here on steemit, but wrote all the above from my memory now. If I could do it so can you (even easier for kids as young as in grade 9).