Euclidean Geometry often has many surprising facts that reveal themselves through careful study.
This one always has been one of my favorites:
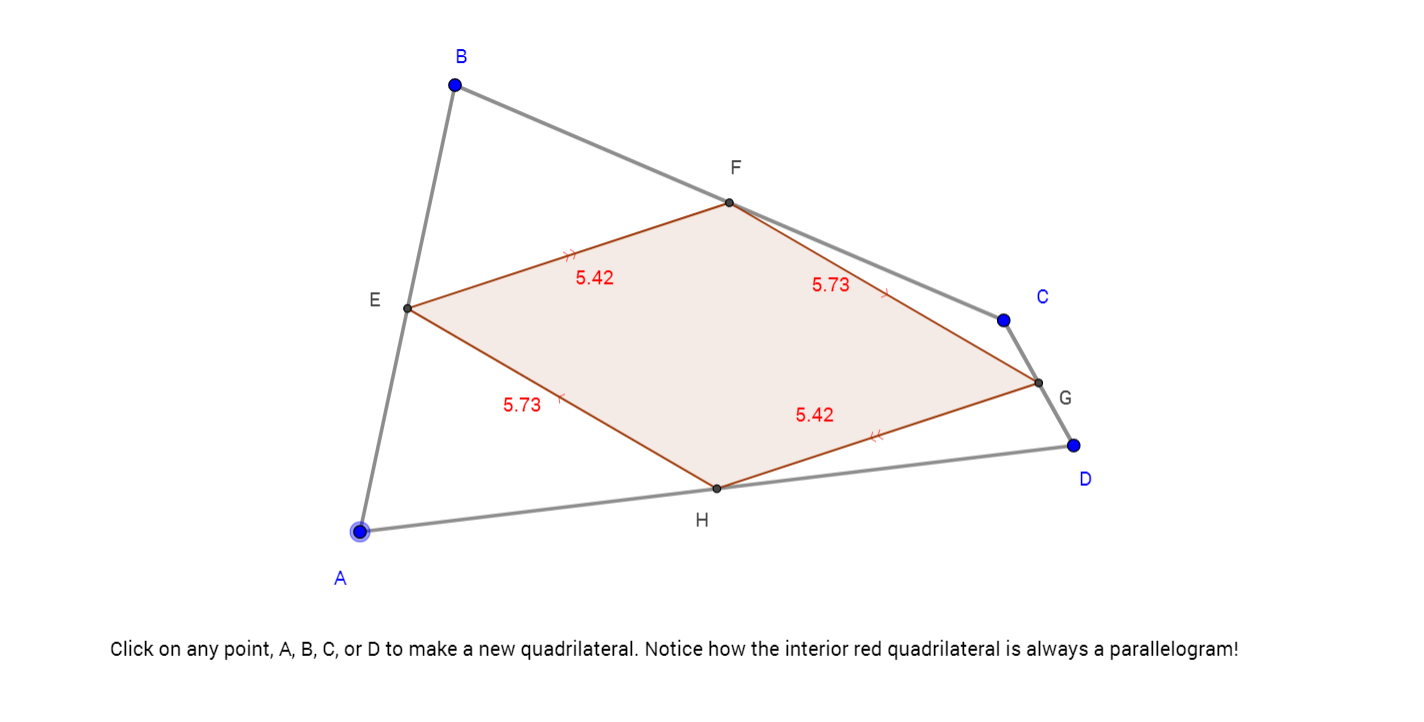
Theorem: The quadrilateral formed by joining the consecutive midpoints of another quadrilateral is a parallelogram.
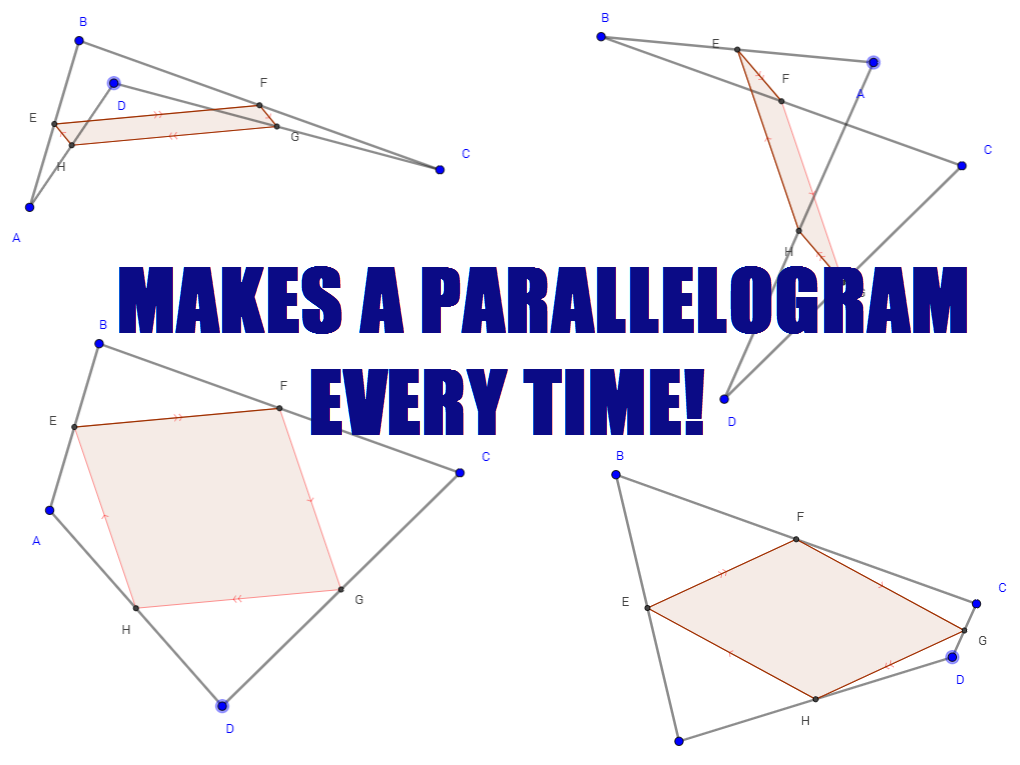
This notion is absolutely stunning. That you can start with any random quadrilateral, convex or concave, and somehow out of it produce another quadrilateral that will always have some order to it.
When I was first learning this, and learning how to teach it, it was in the days of chalkboards and little in the way of interactive instructional tools. Luckily we have software today like Geogebra to assist.
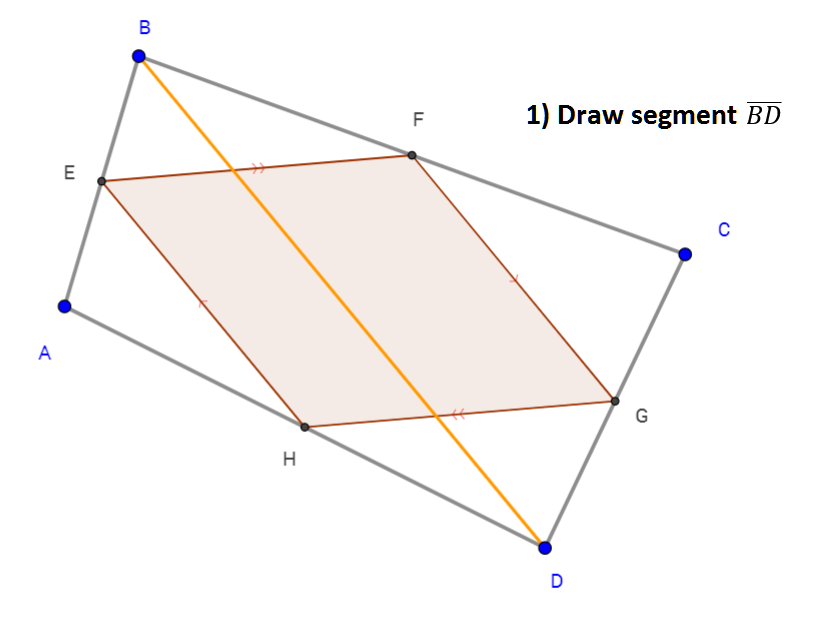
Click on the image below to interact with this quadrilateral!
- You should notice when dragging around points A, B, C, or D that the interior red quadrilateral EFGH is always a parallelogram. The opposite sides are always parallel and congruent.

Want some proof? Oh, I'll give you proof!
But not like the proof you hated doing in your High School Geometry classes. Proofs don't have to be so rigid and boring. They just involve assumptions and logical conclusions from those assumptions.
As an aside, if anyone here on Steemit has a handy guide for combining LaTeX and Markdown, I would be all ears. Having to insert images over and over again is really no fun 😦.
What information is given to us?
- We have a quadrilateral ABCD.
- We have midpoints E, F, G, and H of the segments above,
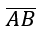 ,
, 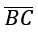 ,
, 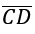 , and
, and  , respectively.
, respectively.
What do we want to show?
- We want to show quadrilateral EFGH is a parallelogram.
To show that EFGH is a parallelogram, we have to first show that their opposite sides are parallel, by definition of a parallelogram. - Or, more formally:
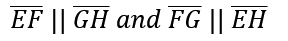
Now, lets show it!
- First a few geometric steps:
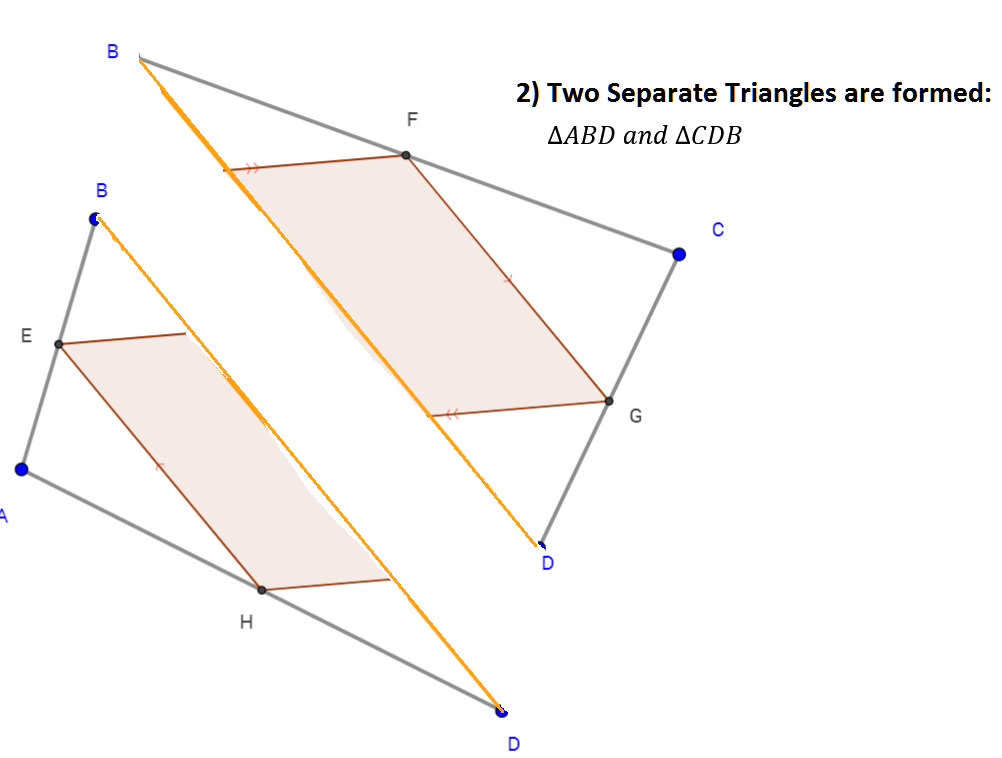
- Now, we draw upon a fact for triangles. Namely, when the midpoints of any two sides are joined to form a line segment, it will always be parallel to the side opposite. This is what is called the Midsegment Theorem.
- So, by the Midsegment Theorem, and the Transitive property, we have the following:
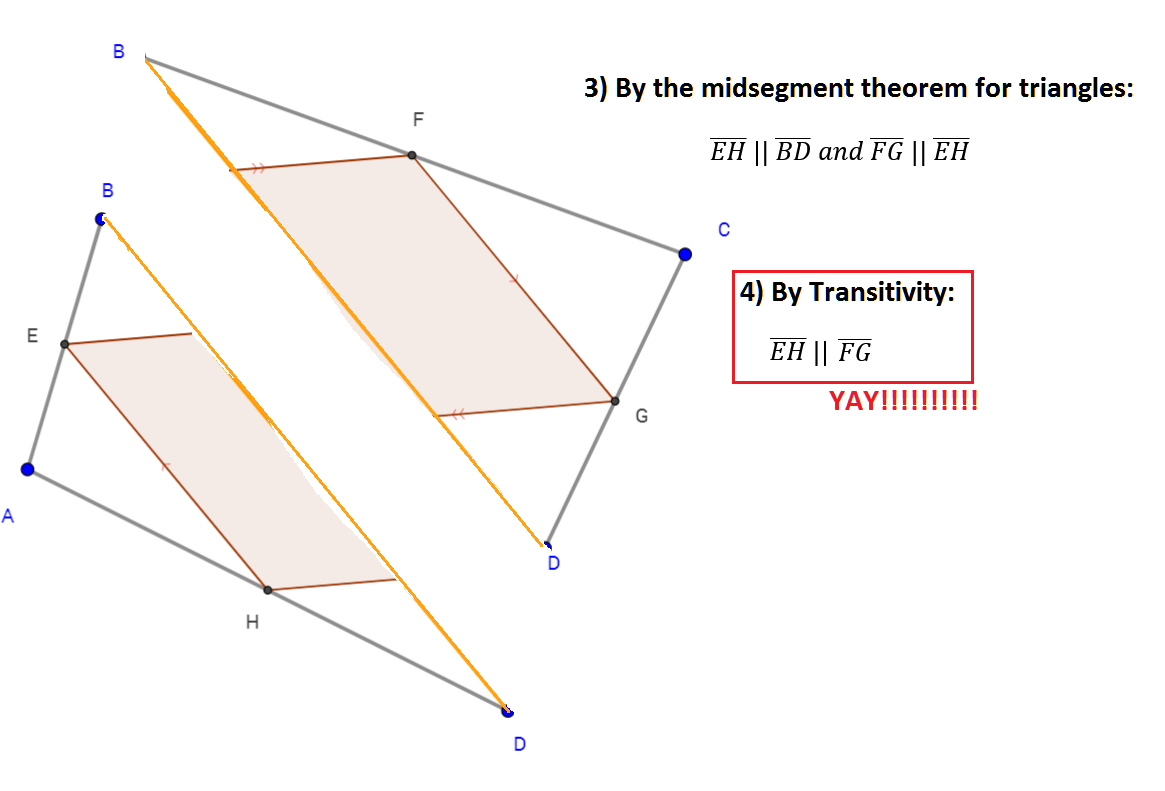
- To show the other two sides are parallel, follow the same series of steps but for a different diagonal.

So, we accomplished what we set out to prove!
Namely, 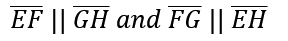
What about the concave polygon?
- It uses the same logic, but this time a diagonal could be outside the shape. Do you see the two triangles that could be formed with the diagonal connecting points A and C?
- I leave it to the reader to connect the dots....bad pun intended ;)
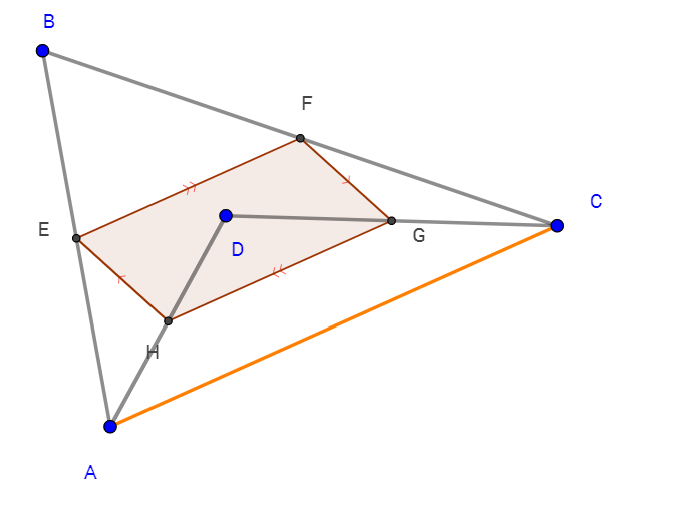
So, armed with some curiosity, and a little bit of elbow grease, we come to a spectacular conclusion.
Often in mathematics, as with life, that's all you need!
Steemit Board Awards - Click Below for More Information