
I still remember that moment in elementary school where after several years and many pages of writing out long division and multiplication, we were finally allowed to use calculators in class. I remember thinking ‘why is this five dollar calculator so much faster than me at this?’ I think it bothered me enough that I asked my teacher that same question. She gave me an answer that even a 10-year-old could tell meant that she didn’t really know. To be fair, my question was definitely outside the realm of elementary school maths. To answer it, we need to look at some principles from neuroscience and physics.
Artificial intelligence has been creeping closer and closer to human performance in recent years. The games of Poker and Go, image recognition, and vocabulary translation are all tasks that AI can now perform better than us. AI is likely to continue gaining on us in the future. However there is no chance that we can ever perform basic maths better than a modern computer. To understand why this is, we need to look at two different kinds of processing: serial processing and parallel processing.

Serial Processing
In serial processing we perform a series of steps one after the other. The next step cannot begin until the previous one has been completed. An example of a task that requires serial processing is getting ready for a shower. This is a task that I mastered years ago, so I understand it well. From experience I have learned that you can’t take off your pants before your shoes, and in fact you need to complete all the undressing activities before getting into the shower. We can represent this process with this diagram.

Serial processing is the basic way that all computers operate, and they have some big advantages over humans at completing tasks that lend themselves to this method. We will go into depth on this point a little later.
In parallel processing we can operate two or more processes at the same time. A good example of this is in the way people process visual information. Take this picture as an example:

If we are sitting in front of this cat, a number of different thought patterns will be going on in our heads. One process will be processing spatial information and recognizing that the cat is rather close to us. Another will be searching through our memory to determine if we know this particular cat. A third will be evaluating the emotion of the cat, with reference to the expression on its face and its posture. We can represent this process with a second diagram:
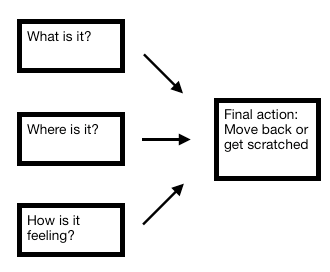
Compared to serial processing we can see that an advantage of parallel processing is that we don’t need to wait to complete one task before starting another.
Let’s go back to my original question about the calculator. Basic maths is a simple, serial operation. In this kind of task, our calculator has two big advantages over us:
- Memory
- Speed
Let’s deal with memory first. When big numbers are involved, basic maths requires us to hold large numbers of digits in our memory. Most people struggle to hold more than seven to nine random digits in our working memory. We can of course write them down, but this takes a bit of time. By comparison, even calculators from the 80s have no problem with working with a couple of 10 digit numbers.

Speed is where it gets interesting. Most people would guess that computers would have a speed advantage over us, but are very likely to underestimate just how big that advantage is. Signals inside our brain can can travel from one neuron to another at the speed of approximately 150 metres per second. Electrical signals inside the processor of a computer can travel at the speed of light- 300,000,000 metres per second. In serial processing we can never compete with something that thinks around 2 million times faster than us. It is a testament to how amazing our brains really are that we are still able to outperform computers at most tasks due to far higher complexity, parallelisation and efficiency
For a detailed explanation of exactly how a calculator converts digits to binary information and performs the calculations check out this excellent article here.
Conclusion
My calculator is far faster than me at basic maths because it has a better memory for random digits, and has a massively faster internal processing speed. Basic maths is a task that requires simple, serial processing-something that computers are great at. Back when humans were still evolving, living in caves, and avoiding tigers, there was no need to regularly work with numbers greater than 10 or so. Our brains are just not designed to perform this kind of task. The flexibility and ability to learn of the human brain has allowed us to learn how to do maths, and to design tools that can perform the task better than us. Just like my elementary school calculator.
In the next article in this series I will discuss how artificial intelligence is starting to learn to ‘think’ in parallel.
