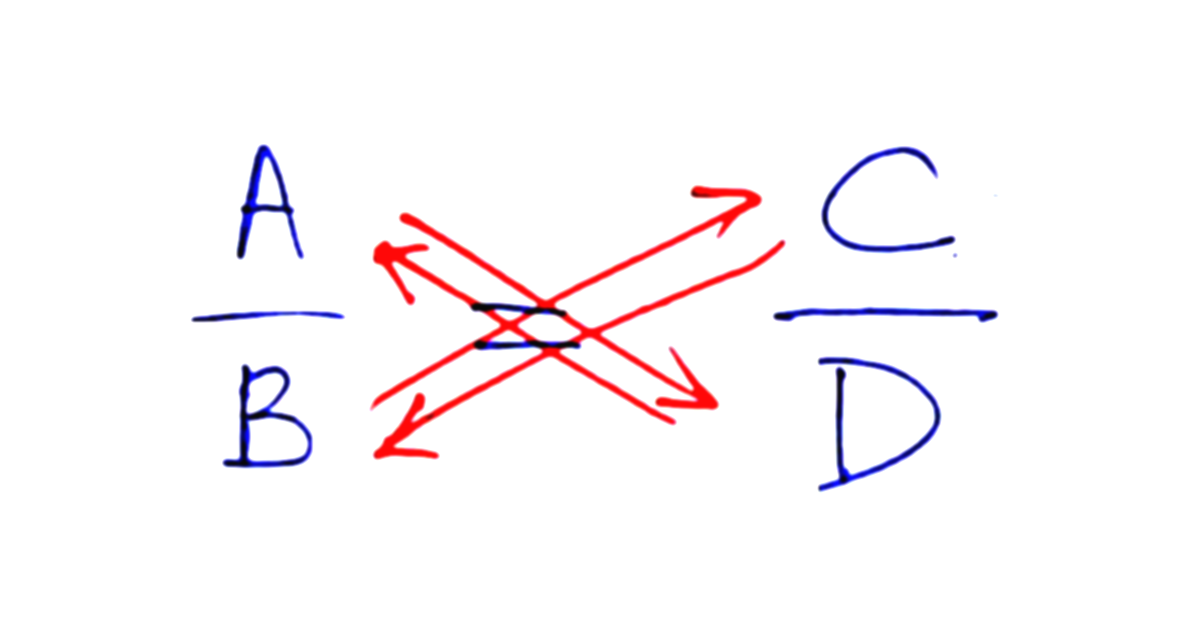
I introduced The Escalator Trick, a very powerful short-cut in
Part 1
and
Part 2
Today, I show you how to melt a "difficult" problem like wax. Take a look at this.
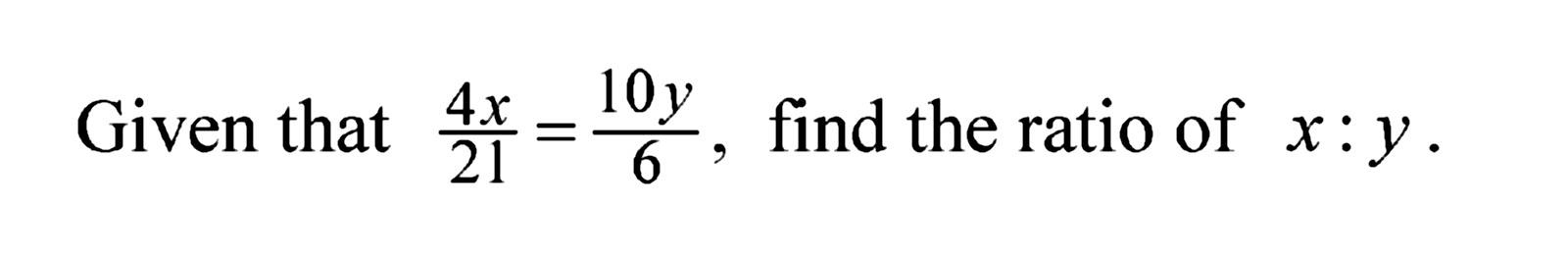
How do you even begin? You can't find your x ('ex') and you don't know y ('why'). Jokes aside, you have only one equation but two unknowns. This is seemingly impossible to solve. However, read the question carefully. It is not asking you to find the individual value of x, nor is it asking you to find the individual value of y. It is asking for the ratio of x:y as if it were one entity. Here is how to do it using the escalator trick.
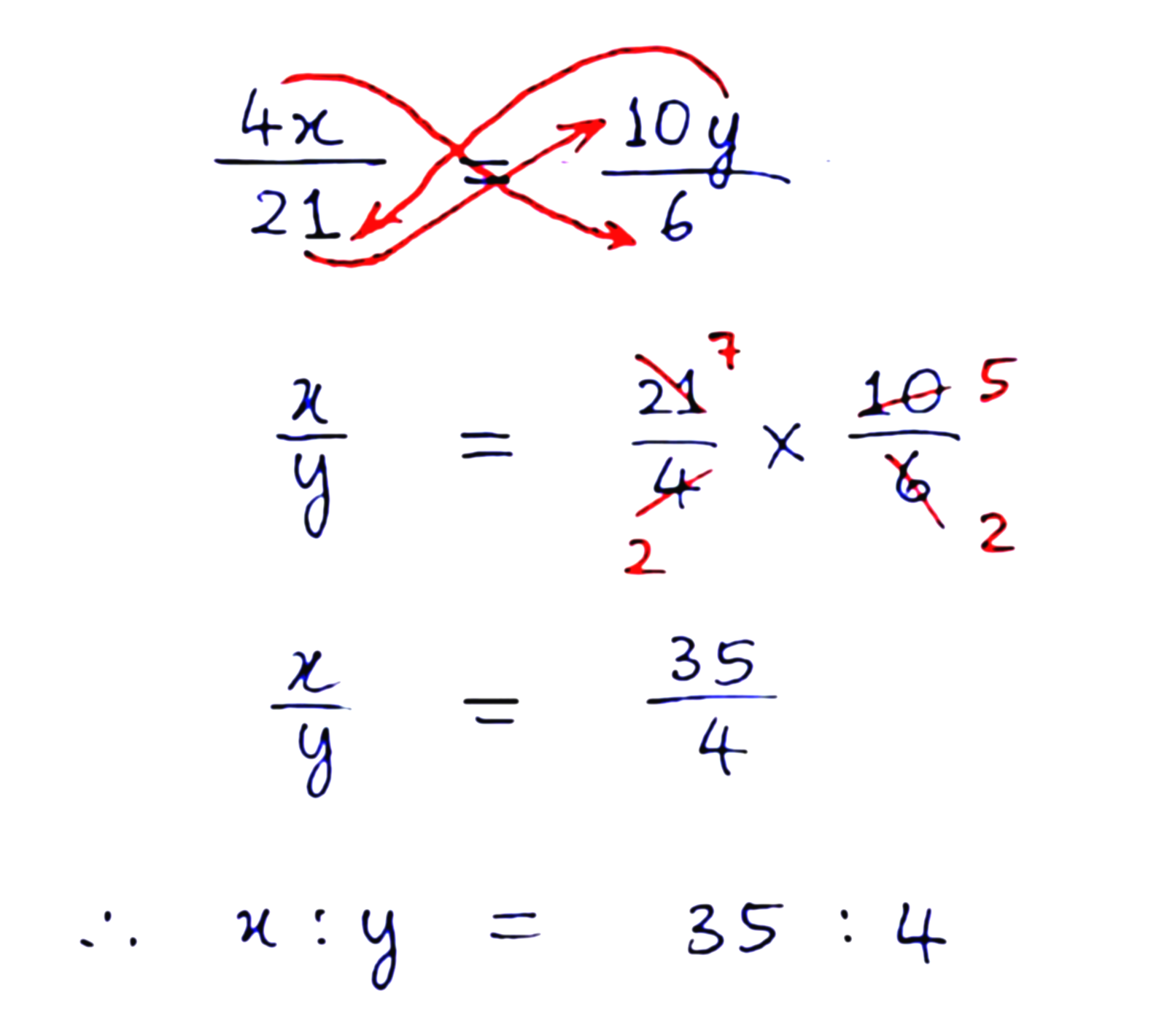
Remember that actually, x:y is sort of a disguise for x/y. We need x and y to be together on the same side, with y appearing below (on the denominator). So pull down the y diagonally across using the escalator. The other stuff that is neither x nor y, namely the 4 and the 21, have to move. So move them diagonally across, which would make them appear as an inverted fraction on the right-hand side. As usual, cancel then simplify. Resist the urge to multiply to get big numbers. Do the cancellations first. We end up with x/y = 35/4, which is just another way of saying x:y = 35:4.
To sum up, the escalator trick is very powerful and useful in situations where the equation to be solved is of the form A/B = C/D.