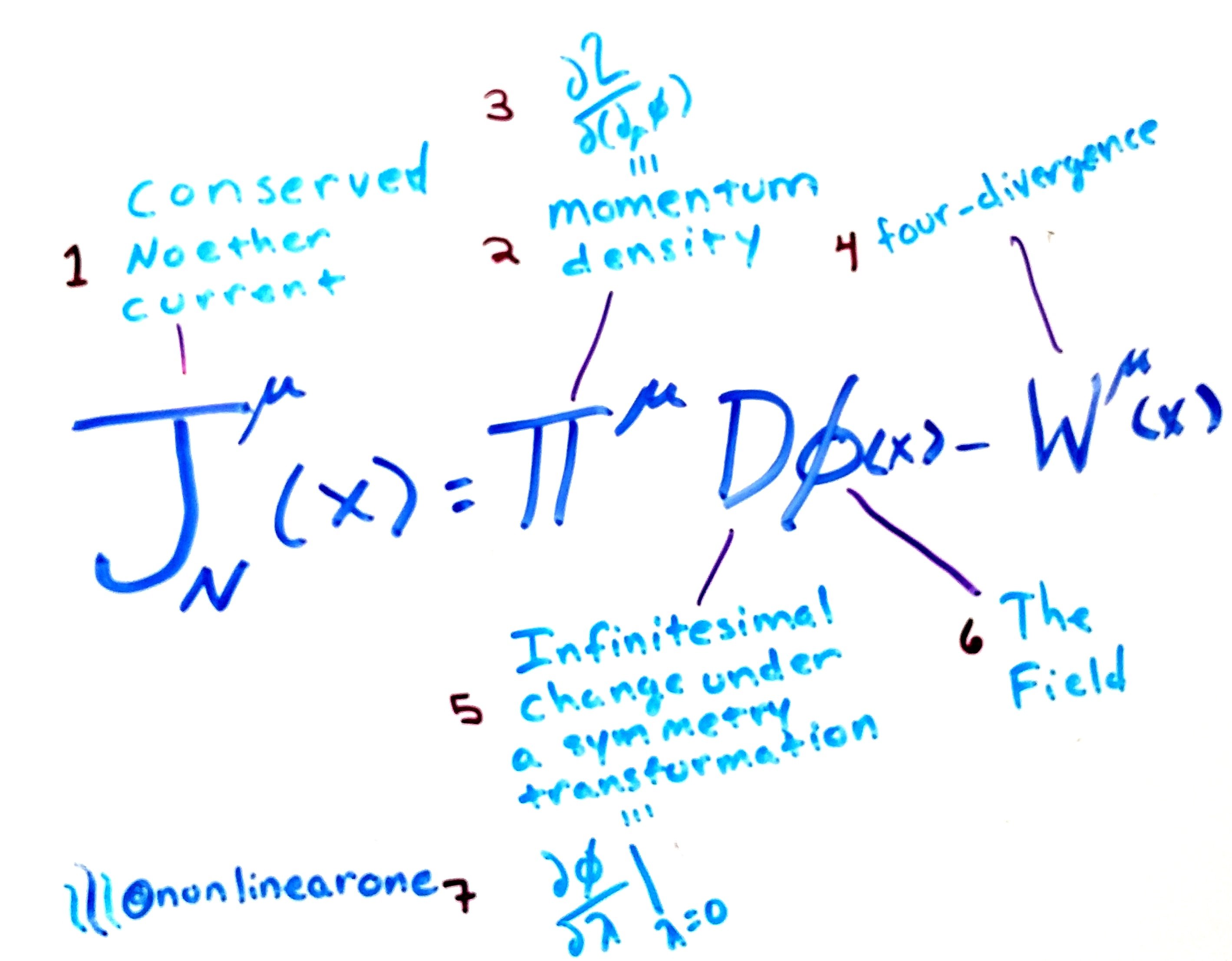
Dr. Bryan here. Your friendly Steem physicist. I think math is art. Physics too (or why else would they have rewarded me with a Master of Arts in Physics?). So, I like to draw equations on the whiteboard. Here's one about the locally conserved Noether Conserved Current (you've probably never heard of this equation, but its among the most important in theoretical physics):
I also like to think of myself as a little teeny bit like Prometheus - I wanna steal the fire from the scientific gods an give it to everybody.
image credit - creative commons
So, in addition to showing the equation in all of its glory (instead of dumbing it all down like everyone else seems to do), I also decided to add annotations to every part of the equation so you can get a glimpse behind the curtain and see what those symbols mean. I'll also post some links to Wikipedia so you can geek out hardcore (read on to find that info).
Noether's (first) Theorem
What's the equation about? It's one of the important equations from Noether's Theorem - basically that symmetries lead to conservation laws.
Noether's (first)[1] theorem states that every differentiable symmetry of the action of a physical system has a corresponding conservation law. The theorem was proven by mathematician Emmy Noether in 1915 and published in 1918.[2] The action of a physical system is the integral over time of a Lagrangian function (which may or may not be an integral over space of a Lagrangian density function), from which the system's behavior can be determined by the principle of least action.
Source: https://en.wikipedia.org/wiki/Noether%27s_theorem
video credit goes to the creator of that video - youtube link
What are those weird u things?
Those are the greek letter u. They mean that the thing they are near is a four-vector (three space coordinates plus a time coordinate).
Annotations
1 - Conserved Noether Current
The formal proof of the theorem utilizes the condition of invariance to derive an expression for a current associated with a conserved physical quantity. In modern (since ca. 1980[4]) terminology, the conserved quantity is called the Noether charge, while the flow carrying that charge is called the Noether current. The Noether current is defined up to a solenoidal (divergenceless) vector field.
Source: https://en.wikipedia.org/wiki/Noether%27s_theorem#Informal_statement_of_the_theorem
2 - Momentum Density
How the Lagrangian Density changes with respect to the space-time variation of the field phi.
image credit: creative commons
3 - Momentum Density Equation
The partial derivative of the Lagrangian Density with respect to the space-time change of the field phi.
4 - Four-Divergence
In differential geometry, the four-gradient ... (or 4-gradient) is the four-vector analogue of the gradient ... from Gibbs-Heaviside vector calculus.
https://en.wikipedia.org/wiki/Four-gradient
5 - Infinitesimal change under a symmetry transformation
Under a symmetry transformation, various quantities will change. One efficient way to describe this is to say that a field phi will change under a symmetry transformation by an amount ... lambda [upside down y looking thing].
Source: Quantum Field Theory for the Gifted Amateur Section 10.1
6 - The Field
Quantum field theory frequently makes use of the Lagrangian formalism from classical field theory. This formalism is analogous to the Lagrangian formalism used in classical mechanics to solve for the motion of a particle under the influence of a field. In classical field theory, one writes down a Lagrangian density (curly L) involving a field, φ(x,t), and possibly its first derivatives (∂φ/∂t and ∇φ), and then applies a field-theoretic form of the Euler–Lagrange equation. Writing coordinates (t, x) = (x0, x1, x2, x3) = xμ, this form of the Euler–Lagrange equation is[10]
https://en.wikipedia.org/wiki/Quantum_field_theory#Lagrangian_formalism
My previous post of the Euler-Lagrange equation (mentioned above)
@nonlinearone/the-euler-lagrange-equation-quantum-field-theory
7 - Symmetry transformation infinitesimal change equation
The Dphi term is shorthand for the partial derivative of phi with respect to lambda evaluated at lambda equals zero.
Still confused?
OK, there is only so much I can do! But ... maybe now that you've seen behind the curtain you can poke around and find out more. There is an amazing wealth of information on Wikipedia!
Google Talk on Emmy Noether and the Fabric of Reality
video credit - the owner of that video on YouTube
p.s. Emmy Noether is the greatest mathematician/scientist you've never heard of. She will be the subject of a future post. Spoiler alert: she is amazing - how many other Jewish female mathematician/physicists do you think there were in Germany at around 1916?
Recommended reading
Quantum Field Theory for the Gifted Amateur. Chapter 10: Symmetry. Section 10.2 Noether's theorem.
