
Where does the Heisenberg uncertainty principle come from?
A quantum particle moves through space in the form of waves and at any moment, the particle might be found at any point on the wave.
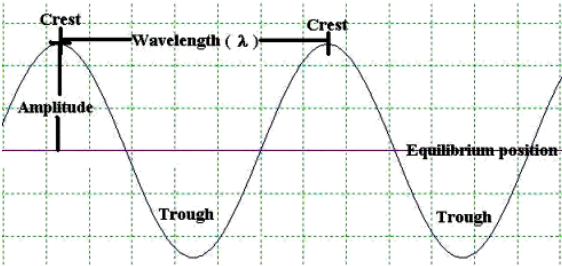
Wave intensity gives the probability of finding a particle.
The shorter the wave, the more certain we are of the particle's position.Momentum is related to wavelength. To determine wavelength accurately, you need an average length of many waves.
The longer the wave, the more certain we are of momentum.
What we need to be more certain about position is the opposite of what we need to be more certain about momentum, and vice versa. Our knowledge is not limited because of our measurement techniques. It is limited because the particle itself is indeterminate.
There might be other uncertainty principles in nature.
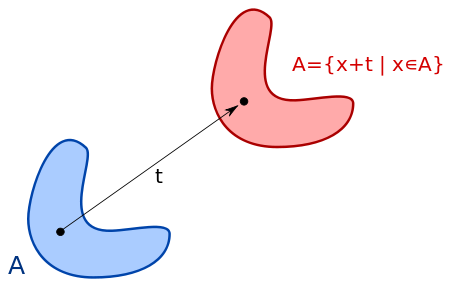
A shift in position can be a symmetry: Shifting everything in x does not change the behavior of x, and p is conserved. There is a similar relationship between time (t) and energy(e). Does this mean there is an uncertainty principle connecting them? Yes, and it involves Plank's Constant. ∆t∆e > h. On the quantum level, we cannot know both time and energy exactly.
Both of these uncertainty principles have many implications in the quantum world.
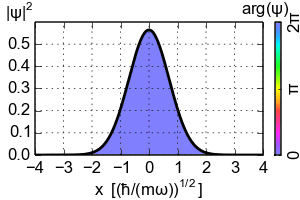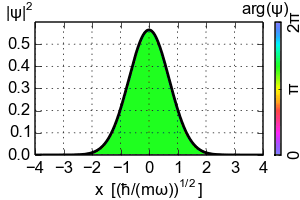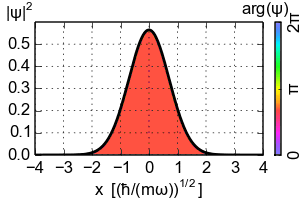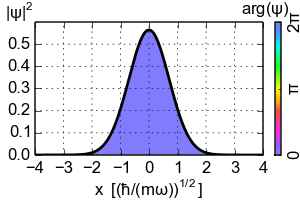
(Zero-point energy
E=ħω/2 causes the ground-state of an harmonic oscillator to advance its phase [color])
An electron exists in a standing wave pattern around a nucleus, confined to a small region of space. Thus its momentum is uncertain. In Newtonian physics, a system can be at rest, with zero energy. But in quantum physics, zero energy would require a particle to have an exact position and momentum, which it cannot have. We call this minimum quantum energy zero-point energy, the random thermal vibrations of atoms even near absolute zero.
Unlike Newtonian physics, quantum mechanics does not allow for a perfect vacuum.

Empty space is really full of an irreducible quantum activity. Quantum theory not only allows but requires the spontaneous appearance of virtual particles in empty space that wink out of existence almost as soon as they appear. A quantum particle can do things that would be impossible for any classical particle, and the implications are amazing.
Uncertain Facts:
- Heisenberg himself was uncertain how to describe his basic idea and often used several different terms: uncertainty, indeterminacy, imprecision, latitude, and statistical spread.

- A stretched wire can vibrate at various frequencies. Such a wire never be exactly at rest, which means that all string instruments have a rest note or frequency which they vibrate at even when not in use.
End Part 5
PART 1, PART 2, PART 3, PART 4, PART 5
Image Credits (in order):
https://static01.nyt.com/images/2016/02/05/business/020816bucks-carl-sketch/020816bucks-carl-sketch-master768.jpghttp://mrknecht.weebly.com/uploads/5/3/3/9/53390171/1432735834.pnghttps://upload.wikimedia.org/wikipedia/commons/thumb/9/91/Translation_of_a_set.svg/450px-Translation_of_a_set.svg.pnghttps://upload.wikimedia.org/wikipedia/commons/2/2f/QHO-groundstate-animation-color.gifhttps://thumbs.mic.com/NGVlMmQ2NWYzYiMvcXlMcmliZjQ0SlpNUGZJQl9iLTc5MnBDUW1VPS9maXQtaW4vOTAweDkwMC9maWx0ZXJzOm5vX3Vwc2NhbGUoKTpxdWFsaXR5KDgwKS9odHRwOi8vaW1hZ2VzLm1pYy5jb20vY2VwZmx1bmx3cWFhbW9qdzZrbTZzdXdodHpjcWltdmg3dG1seGd6MHpiajJ6cHFpOGhpZ2Y2dmc2cjZ1ZXp6ZS5naWY.gifhttps://upload.wikimedia.org/wikipedia/en/thumb/6/6f/Bohr_heisen_pauli.jpg/330px-Bohr_heisen_pauli.jpghttp://i.imgur.com/owzMkQF.gif
