
After a week of a well-deserved break, I am now back on tracks to discuss physics again.
Instead of chatting about particle physics or cosmology, I decided this time to move on with my series of posts on quantum mechanics. To make a long story short, those are connected to lectures I have held for bachelor students a couple of years ago. The table of content is available below.
With this 9th post on the topic (and the next one), I will discuss old models trying to explain the atomic structure.
Those are good examples of the scientific method. Theories have been designed to explain what was going on with atoms, in particular the planetary model of Rutherford (this post) and the model of Bohr (the next post). But data started to push a little bit later in favor of an alternative theory (i.e. quantum mechanics) and both Rutherford and Bohr’s model are now lying in history of science books.
It is however instructive to understand how these models work and what went wrong with them. They also contributed a lot to the early developments of quantum mechanics, which is why they are important to discuss.
THE PLANETARY MODEL OF THE ATOM IN A NUTSHELL

[image credits: Wikipedia]
The atomic nucleus was discovered in the beginning of the 20th century by Rutherford, who immediately tried to propose a model explaining the observations.
Its first attempt was introduced around the year 1911, and is known as the planetary model of the atom. This model is illustrated on the picture on the right.
The name is very easy to understand and stems from our own solar system. The latter is constituted of a central sun around which planets orbit. The atom was suggested to work the same way:
- One has a central positive charge (that is known called the atomic nucleus);
- One has a bunch of orbiting electrons around this central charge.
The central charge is assimilated to the sun and the orbiting electrons to planets. This can in fact easily be imagined from the above picture.
I recall that particles with an opposite electric charges attract each other. A negatively charged electron should hence be attracted by the nucleus and collapse with it at some point. However, the high velocity of the electron is saving us by compensating the electromagnetic forces.
THE THEORY FRAMEWORK - THE CASE OF HYDROGEN
The theoretical context around Rutherford model is based on classical mechanics. By the way, a picture of Rutherford is shown on the left. Better now than never to introduce the guy.

[image credits: Nobel Prize]
Instead of making things complicated from the start, let us pick up one example: the simplest atom ever, hydrogen.
The hydrogen atom is made of a single proton (the nucleus) and a single electron orbiting around it. The atomic nucleus contains thus a single particle (for the sake of the comparison, it is interesting to mention that uranium nuclei contain about 235 entities and is thus way more complicated).
In other words, the hydrogen atom can thus be seen as a two-body system constituted of a central proton and a single orbiting electron. A bit like Earth and the moon, except that the two particles are here interacting via Coulomb interactions.
The idea that the proton is fixed and that the electron is orbiting around it is not crazy. One may naively think that the proton could orbit around the electron, or that both bodies could have complicated motions around each other (like Pluto and Charon in the planet analogy).
The electron mass is however almost 2000 times smaller than the proton mass. As a result, it makes total sense to assume that the proton is almost at rest and that the electron is the guy moving at high velocity around it.
The equations then stem from the basic principles of dynamics.
BASIC CLASSICAL MECHANICS AND THE HYDROGEN ATOM
In order to derive the equations behind the motion of the electron and to predict the energy spectrum of the hydrogen atom, Rutherford model is based on classical mechanics (and thus very basic principles).
In order to write what is going on for the electron, we use Newton’s law. The electron mass times the electron acceleration equals the sum of the forces applied on the electron, once we consider a Galilean reference frame with the fixed proton at the origin.
There is only a single force in the game: the Coulomb interaction of the electron with the proton. This can be translated in equation as follows,
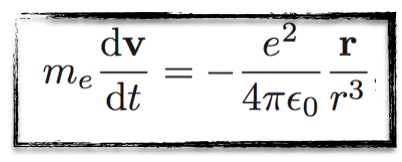
I promise, I will restrain myself with the number of equations in this post, but it is good to show at least one (or two, see below) of them for the fun… :)
The above equation is kind of easy to understand.
On the left hand side, we have the product of the electron mass (me) and the electron acceleration (that is the temporal derivative of the electron velocity v).
On the right-hand side, we get the expression of the Coulomb interaction between the electron and the proton. Except a bunch of constants, the important quantity is the position of the electron r and its norm r.
This may sound complicated, but it is not. This is a very well-known problem in mechanics.
I will skip any details, but the main result is that for a bound states, the trajectories are ellipses. In other words, when the proton and the electron form a composite object (that is the atom that we are after), the electron will follow an elliptic trajectory around the proton.
The distance between the electron and the proton can be simply derived and depends on the electron velocity,. If the velocity is constant (which can be a good approximation for a given period of time), the trajectory is thus circular and centered on the proton.
THE HYDROGEN ENERGY SPECTRUM
[image credits: UNL]
In order to simplify the above picture, we can assume that the velocity of the electron is constant (as just mentioned above). In this case, electron trajectories are circular, again as said above.
The reason I want to make this approximation is that it allows to get easily the energy spectrum of the atom of hydrogen.
Defining, as usual, the total energy as the sum of the potential energy (related to the Coulomb interaction between the electron and the positron) and the kinetic energy of the electron, one gets

The important point is that for each radius, we get a well defined energy (in a circle, the radius is constant). Or vice versa.
AND ELECTROMAGNETISM NOW KILLS THE MODEL
And now comes the problem. It has a very simple name: electromagnetism.
Electromagnetism tells us that any accelerated charged particle radiates energy. This is observed, e.g., at electron-positron collider where the accelerated particles radiate electromagnetic energy before colliding.
Therefore, an accelerated electron orbiting around a nucleus radiates energy, so that its total energy is getting more and more reduced. After a while, the electron would thus collide with the nucleus.
As a consequence, the hydrogen atom cannot be stable in the atomic planetary model. And this is a big deal: Rutherford model is hence wrong.
SUMMARY AND REFERENCES
In this post, I continued my introduction to the path that has led to quantum mechanics. The hydrogen atom and the explanation of its properties have played a key role with this respect.
One of the first model proposed to explain it was introduced by Rutherford, and is named the planetary model of the atom. Similarly to what is going on in the solar system, electrons are seen as planet orbiting around the atomic nucleus, that is itself seen as a kind of sun.
This model was however wrong, due to electromagnetism. This indeed would have implied that the hydrogen atom cannot be stable (which contradicts observations, of course). In my next post, I will detail what Bohr did in 1913 to generalize this model and make it working.
For more information, I suggest to read the Wikipedia page on the Rutherford atomic model. Or from any lecture note that could be found on the web ;)
Table of contents of this series of posts
I. Introduction
1. Concepts and fundations
2. Interactions and conservation laws
3. Systems of particles
II. The origins of quantum mechanics
1. Fundamental physics at the beginning of the 20th century
2. The mysteries of the atomic spectra
3. The mysteries of the black bodies
4. Quantization of the electromagnetic radiation and the birth of the photons
5. The Compton effect
6. The planetary model of the atom (this post)
